Family Of Circles

In this page we are going to discuss about family of circles.Here concentric circles plays a major role.First let us consider the definition of concentric circles.Two or more circles having same center is called the concentric circles.There are two types in family of circles.

Circles touching each other:

Two circles may touch each other either internally or externally.

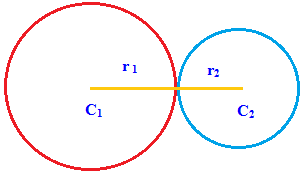
Circles touching each other externally:

Here the center of two circles are C₁ and C₂ and radius are r₁ and r₂.The distance between their centers is sum of their radii.

If the two circles are touching each other externally then they must satisfy the following condition.

  C₁ C₂ = r₁ + r₂

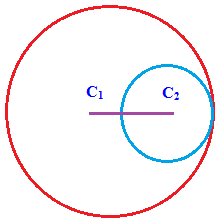
Circles touching each other internally:

Here the center of two circles are C₁ and C₂ and radius are r₁ and r₂.The distance between their centers is differences of their radii.

If the two circles are touching each other internally then they must satisfy the following condition.

  C₁  C₂ = r₁ - r₂

Example 1:

Show that the circles x² + y² -4x + 6y + 8 = 0 and x² + y² -10x - 6y + 14 = 0 touch each other.Find whether two circles are in which family of the circles/.  

Solution:

First let us find the centers of two circles

Center of any circle will be (-g,-f). So first let us compare the first equation with the general form of circle to get the value of g and f.

x² + y² -4x + 6y + 8 = 0

x² + y² + 2g₁x + 2f₁y + c = 0

Here 2g₁ = -4  2f₁ = 6  and c₁ = 8

       g₁ = -2     f₁ = 3

Center of the first circle (2,-3)

Now let us compare the second equation with the general form to get the values of g and f

x² + y² -10x - 6y + 14 = 0

Here 2g₂ = -10   2f₂ = -6 and c₂ = 14

        g₂ = -5      f₂ = -3

Center of the second circle (5,3)

So C₁ is (2,-3) and C₂ is (5,3)

Distance between C₁C₂ = √(5-2)² + (3+3)²

                               = √3² + 6² 

                              = √9 + 36

                              = √45

                              = 3√5    ----------(1)

Now let us find r₁

                         r₁ = √g₁² + f₁² - c₁

                           = √(-2)² + 3² - 8

                           = √4 + 9 - 8

                           =  √5

Now let us find r₂

                         r₂ = √g₂² + f₂² - c₂

                           = √(-5)² + (-3)² - 14

                           = √25 + 9 - 14

                           =  √34-14

                           =  √20

                           =  2√5

              r₁ + r₂ = √5 + 2√5

                       =  3√5 --------(2)

Since (1) and (2) are equal we can say each circles touch each other externally.