Centroid Of The Triangle

In this page centroid of the triangle we are going to see the formula to find the centroid of any triangle. To find centroid we must given three vertices of the triangle.First let us see the definition of centroid.

Definition:

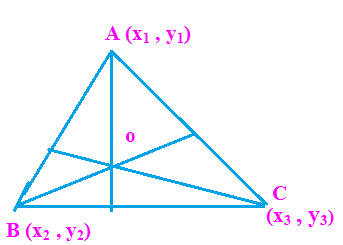
There are three medians of the triangle and they are concurrent at a point O,that point is called the centroid of a triangle.

In the following diagram O is the centroid of ABC.Now let us look into the formula.

Centroid of a triangle = (x1+x2+x3)/3, (y1+y2+y3)/3

Example 1:

Find the centroid of a triangle whose vertices are the points (8,4)(1,3) and (3,-1).

Solution:

Centroid of a triangle is

Centroid of a triangle = (x1+x2+x3)/3, (y1+y2+y3)/3

Here we have x1 = 8, x2 = 1and x3 = 3

                   y1 = 4, y2 = 3 and y3 = -1

                                  = (8 + 2 + 3)/3 , (4 + 3 -1)/3

                                  = (12/3) , (6/3)

                                  = (4,2)

Therefore the centroid is (4,2)

---

Example 2:

If a triangle has its centroid at (4,3) and two of its vertices are (2,-1) and (7,8),find the third vertex.

Solution:

Let(a,b) be the required vertex.So the vertices of the triangle are (2,-1) (7,8) and (a,b) and the centroid is (4,3)

here x1 = 2,x2 = 7, x3 = a,y1 = -1,y2 = 8 and y3 = b  

By applying the formula

               Centroid  = (x1+x2+x3)/3, (y1+y2+y3)/3

  By applying the formula

                           (4,3)  =  (2+7+a)/3 ,(-1+8+b)

                           (4,3)  = (9+a)/3 ,(7+b)/3

                       (9+a)/3 = 4            (7+b)/3 = 3

                    9 + a = 4 x 3              7 + b = 3 x 3

                     9 + a = 12                 7 + b = 9

                          a = 12-9                 b = 9-7

                          a = 3                     b = 2

Therefore the required vertex is (3,2)